Magnetic topological insulators (MTIs) display a fascinating interplay between magnetic order and topologically nontrivial band structures. In the MAGMA project (“Magnetic topological insulators for robust Majorana bound states”), which is funded by the European QuantERA program and by the national research agencies of Luxembourg, Germany, and Spain, we will investigate MTI nanostructures experimentally and theoretically, in particular in the presence of superconductivity induced by the proximity effect. The aim of this project is to engineer Majorana bound states and to establish MTIs as a platform for topological quantum computation.
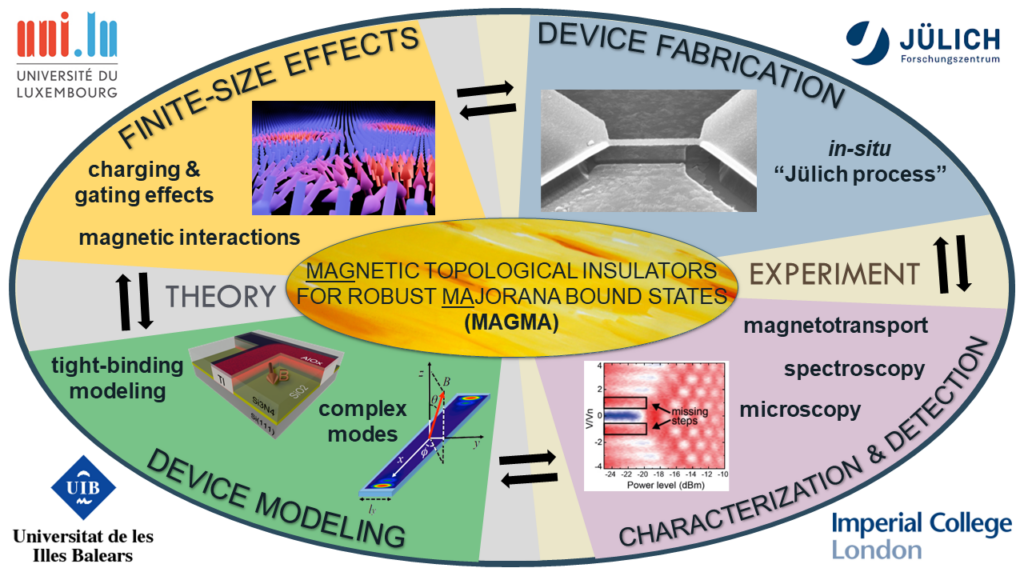
The experimental work will be performed at Forschungszentrum Jülich (FZJ) and will involve device fabrication using electron beam lithography and molecular beam epitaxy, as well as device characterization by magnetotransport measurements and scanning probe measurements in collaboration with Imperial College London (ICL). The theory work will be performed at the University of Luxembourg (UL), the University of the Balearic Islands (UIB) and FZJ, and will consist in studying finite-size effects in MTIs using analytical and numerical methods, as well as hybrid-device modelling using complex-band-structure techniques and tight-binding simulations.
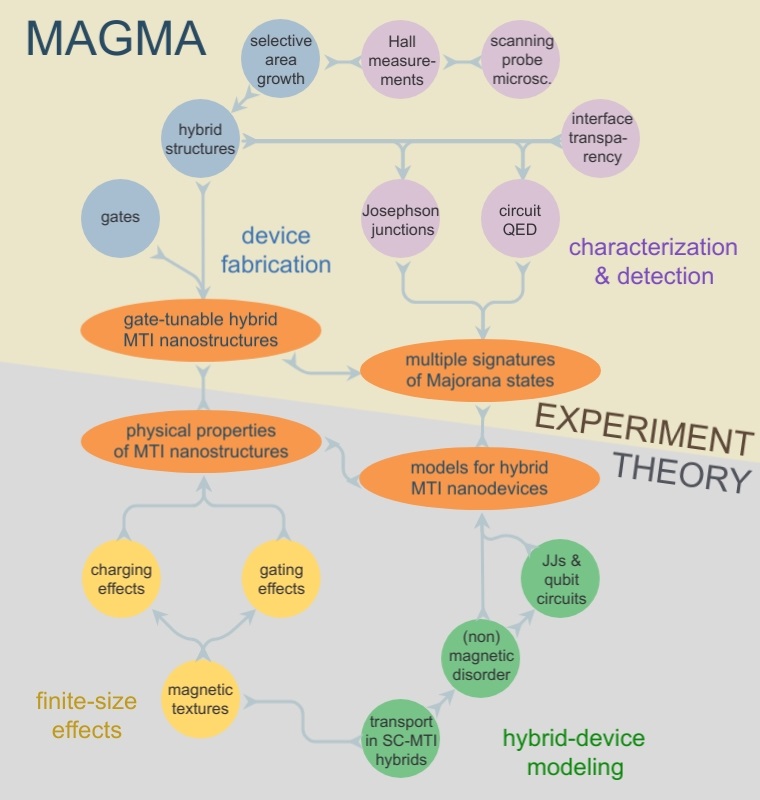
The unique experimental platform available within this consortium as well as its theoretical know-how are ideally suited to address this challenge. On the experimental side, we will construct magnetic topological insulator (MTI) nanostructures in situ in different device geometries, e.g., as nanoribbons, junctions, or interferometers. Magneto-transport measurements will allow us a detailed characterization of the devices and their topological properties in the normal-conducting state. Next, we will apply superconducting leads for studying proximity-induced superconductivity and Josephson junctions based on MTIs. This finally will make it possible to create and detect Majorana bound states based on MTIs.
The techniques used by our theory and simulation cluster will allow multiscale modeling of nanostructure-based devices and will closely accompany the experimental efforts. A constant feedback loop between experiments and theory will help us optimize device geometries and materials. While bulk MTIs have been investigated in detail, their nanostructures still leave open many fundamental questions. This project will therefore lead to important new insights, both in theory and experiment, about MTIs. The latter, in turn, will lead us to the ultimate goal of our project, which is to unambiguously identify Majorana bound states in MTI nanoribbons, and to pave the way for their future use in topological quantum computation.